Multiple Choice
Identify the
choice that best completes the statement or answers the question.
|
|
|
1.
|
Use this balance-scales model to solve for x. 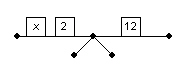
|
|
|
2.
|
In the left pan of a set of balance-scales, there are 2 identical unknown masses
and a mass of 18 g.
The scales are balanced by adding a mass of 24 g to the right pan.
Find
the value of each unknown mass.
|
|
|
3.
|
Write an equation for this sentence.
40 less than a number is 10.
|
|
|
4.
|
Solve this equation. 14 – 2x = 6
|
|
|
5.
|
Write an equation for this sentence.
A number divided by 4 is 11.
|
|
|
6.
|
Expand. 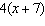 a. | 4x + 7 | b. | 4x + 28 | c. | 4 + x + 7 | d. | 28x |
|
|
|
7.
|
Solve this equation: 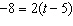
|
|
|
8.
|
The price of an electronic puzzle was reduced by $7.
Mr. Murray bought 10
puzzles for his relatives. The total cost before taxes was $130.
What was the original price of
the puzzle?
a. | $7.65 | b. | $20.00 | c. | $6.00 | d. | $127.00 |
|
|
|
9.
|
Doreen chose an integer. She added 4, then multiplied the sum by –3.
The product was –15. What integer did Doreen start with?
a. | –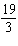 | b. | –1 | c. | 1 | d. | 0 |
|
|
|
10.
|
Complete this table of values for the relation 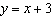 . 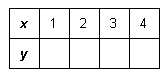
|
|
|
11.
|
Complete this table of values for the relation 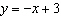 . 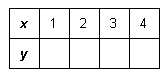
|
|
|
12.
|
Complete this table of values for the relation 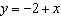 . 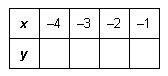
|
|
|
13.
|
Make a table of values for the relation 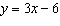 for x = 1, 2, 3,
and 4.
|
|
|
14.
|
The ordered pair ( , 5) is in the linear relation with equation  .
Find the missing number in the ordered pair. a. | 3 | b. | 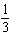 | c. | 6 | d. | –6 |
|
|
|
15.
|
Describe the relationship between the variables x and y in this
graph. Graph of 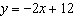  a. | When x increases by 1, y decreases by 12. | b. | When x
increases by 1, y decreases by 2. | c. | When x increases by 1, y
increases by 2. | d. | When x increases by 2, y increases by
12. |
|
Short Answer
|
|
|
16.
|
Use this balance-scales model to solve for x. 
|
|
|
17.
|
A set of balance scales has an unknown mass and a mass of 4 g in its left
pan.
The scales are balanced by adding a mass of 21 g to the right pan.
Find the unknown
mass.
|
|
|
18.
|
What is the mass needed to balance these scales? 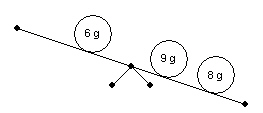
|
|
|
19.
|
Solve this equation. 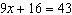
|
|
|
20.
|
Solve this equation. 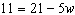
|
|
|
21.
|
Jacqui has $56 in her savings account. She saves $25 each week.
How long
will it take before she has $406 in her account?
Write an equation, then solve the problem.
|
|
|
22.
|
Solve this equation. 
|
|
|
23.
|
Which value, 4, 6, or 8, is the correct solution to the equation 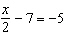 ?
Explain.
|
|
|
24.
|
Expand. 
|
|
|
25.
|
This hexagon has a perimeter of 52 cm. What is the length of the side
marked a? 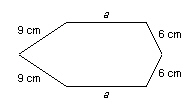 a) Write an equation to find the length of
side a that involves the distributive property.
b) Solve the
equation and answer the question.
|
|
|
26.
|
Solve this equation: 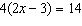
|
|
|
27.
|
The equation of a linear relation is 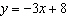 . Which ordered pairs are NOT in the
relation? A(3, –1), B(7, –13), C(8, –16), D(9, –20)
|
|
|
28.
|
Iris has $240 in the bank and she withdraws $25 every week. An equation for
this relation is 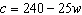 , where c represents the amount of money, in
dollars, in Iris’ account and w represents the number of weeks. How much does Iris
have in her account after 4 weeks?
|
|
|
29.
|
This table of values is for the linear relation with equation 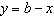 , where
b is a constant. Find the value of b. 
|
|
|
30.
|
This graph shows the linear relation  . Describe the relationship between
the variables x and y. 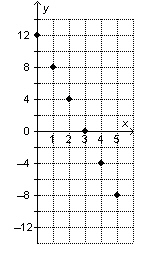
|
Problem
|
|
|
31.
|
If a star has a value of 26, what is the value of the happy face? What
can you say about the value of the diamond shape?
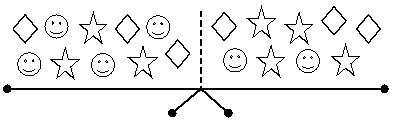
|
|
|
32.
|
Expand.  Show your work.
|
|
|
33.
|
a) Write a formula
for the perimeter, P, of this hexagon 2 different ways.
b)
Find the perimeter of the hexagon when a = 4 cm and b = 8 cm.
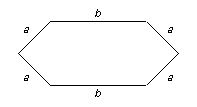
|
|
|
34.
|
Simplify this expression. 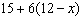 Show your work.
|
|
|
35.
|
Carmel used the distributive property to solve this equation: 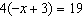 Here’s her work. 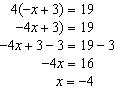 a) Check Carmel’s work. Is her solution
correct?
b) If your answer is yes, verify the solution. If your
answer is no, describe the error, then correct it.
|
|
|
36.
|
The length of a rectangle is 5 cm more than its
width.
a) Write a formula for the perimeter, P, of the
rectangle that involves the distributive property.
b) Find the
length and width of the rectangle if the perimeter is 58 cm. Show your work.
|
|
|
37.
|
Make a table of values for the relations  and  . For what
value of x will the relations have the same y-value?
|
|
|
38.
|
Make a table of values for the relation  for integer values of
x from –3 to 3. a) Find
the value of y when x = 7.
b) Find the value of
x when y = 7.
c) Estimate the value of y when
x = 1.5.
|
|
|
39.
|
Barb makes and sells calendars. The cost of making a batch of calendars is
$1050 and Barb can sell each calendar for $7. If s represent the number of calendars sold
and p represents Barb’s profit in dollars, an equation for this relation is 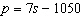 . a) How many calendars
does Barb have to sell to cover the cost of making a batch of
calendars?
b) What is Barb’s profit if she sells 180 of the
batch of calendars?
Show your work.
|
|
|
40.
|
a) Graph both
relations on the same coordinate axes.
i) 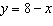
ii) 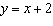 b) Find the ordered
pair on the graph that are in both relations.
|